Simple Harmonic Motion (SHM) is a fundamental concept in IB Physics that appears under the topic of oscillations. It’s a beautiful dance of physics where forces and motion interplay harmoniously. For IB students, mastering SHM isn’t just about memorizing formulas—it’s about understanding the underlying principles that govern oscillatory motion. Our IB Physics tutor broke the concept of SHM down into bite-sized chunks to make it both digestible and interesting.
What Is Simple Harmonic Motion?
Simple Harmonic Motion (SHM) refers to a type of periodic motion where an object moves back and forth along a path, returning to its equilibrium position. Think of a swinging pendulum or a vibrating guitar string—they follow SHM.
At its core, SHM arises when a restoring force acts on the object, trying to bring it back to its equilibrium position. The restoring force is directly proportional to the displacement but acts in the opposite direction.
Mathematically, this is expressed as:
F = -kxWhere:
- F is the restoring force.
- k is the force constant or spring constant (a measure of stiffness).
- x is the displacement from equilibrium.
The negative sign indicates that the force opposes the displacement.
Key Characteristics of Simple Harmonic Motion
To grasp SHM, you must familiarize yourself with its unique traits:
- Oscillatory Nature: SHM involves motion that repeats itself in regular intervals (periodic motion).
- Equilibrium Position: The midpoint where the object rests when undisturbed.
- Restoring Force: A force proportional to displacement, always aiming to restore equilibrium.
- Sinusoidal Motion: The displacement, velocity, and acceleration graphs of SHM follow sine or cosine curves.
Real-World Examples of Simple Harmonic Motion
- A mass attached to a spring.
- A child on a swing.
- Vibrations of molecules in solids.
SHM and Its Mathematical Foundation
Let’s break down the math step by step.
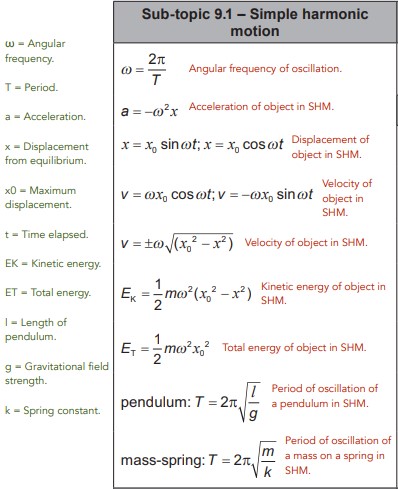
Simple Harmonic Motion IB Physics Formula Booklet Annotated by ibphysics.org
Displacement in Simple Harmonic Motion
The displacement of an object in SHM can be modeled as:
x(t) = A \cos(\omega t + \phi)Where:
- A is the amplitude (maximum displacement).
- \omega is the angular frequency.
- t is time.
- \phi is the phase constant (determines the starting point of the motion).
Velocity in Simple Harmonic Motion
Velocity is the rate of change of displacement:
v(t) = -A \omega \sin(\omega t + \phi)Notice that velocity reaches its maximum when the object passes through equilibrium and zero at the maximum displacement.
Acceleration in SHM
Acceleration is the rate of change of velocity and is proportional to displacement:
a(t) = -\omega^2 x(t)Here, a(t) is always directed towards the equilibrium position.
Period and Frequency of Simple Harmonic Motion
In SHM, period (T) and frequency (f) describe how quickly the motion repeats.
Period: The time taken for one complete oscillation.
T = \frac{2\pi}{\omega}Frequency: The number of oscillations per second.
f = \frac{1}{T}Angular Frequency:
\omega = 2\pi f
Energy in Simple Harmonic Motion
Energy in SHM is constantly exchanged between potential and kinetic energy. Here’s how:
Potential Energy (PE):
PE = \frac{1}{2}kx^2
Maximum at maximum displacement.Kinetic Energy (KE):
KE = \frac{1}{2}mv^2
Maximum at equilibrium.Total Energy (E):
E = KE + PE = \frac{1}{2}kA^2
Energy remains constant throughout the motion.
Phase Relationships in SHM
Understanding how displacement, velocity, and acceleration relate in SHM is crucial. Here’s a summary:
| Quantity | Phase Relationship | Max Value |
|---|---|---|
| Displacement | x(t) | A |
| Velocity | v(t) = -A \omega \sin(\omega t) | A\omega |
| Acceleration | a(t) = -\omega^2 x(t) | A\omega^2 |
Common SHM Scenarios in IB Physics
Mass-Spring System
For a spring-mass system:
Angular frequency:
\omega = \sqrt{\frac{k}{m}}Period:
T = 2\pi \sqrt{\frac{m}{k}}
Simple Pendulum
For a pendulum (small angle approximation):
Angular frequency:
\omega = \sqrt{\frac{g}{l}}Period:
T = 2\pi \sqrt{\frac{l}{g}}
Where:
- g is the acceleration due to gravity.
- l is the length of the pendulum.
Practical Tips for IB Physics Students
- Visualize the Motion: Sketch the displacement, velocity, and acceleration graphs to see how they interrelate.
- Memorize Key Formulas: Focus on angular frequency, period, and energy equations.
- Link to Real-Life Examples: Relate SHM concepts to pendulums, springs, and vibrations to strengthen understanding.
- Practice Problems: Solve IB past paper questions to gain confidence.
Conclusion
Simple Harmonic Motion is a cornerstone of physics, offering insights into oscillatory phenomena. By understanding its core principles, students can connect mathematical formulas to physical systems. Whether it’s a pendulum ticking away or a spring bouncing, SHM brings physics to life.
FAQ
SHM models many natural phenomena, from atomic vibrations to engineering systems. It helps us predict and analyze oscillatory behavior.
SHM specifically involves a restoring force proportional to displacement, whereas periodic motion may not.
Look for systems with equilibrium positions and forces that increase as displacement grows.
Damping is a force that reduces the amplitude of oscillations over time, like friction or air resistance.
No, the restoring force is essential for SHM. Without it, the motion cannot return to equilibrium.