Geometric sequences and series are an essential topic in the International Baccalaureate (IB) Math curriculum. Geometric sequences and series are a type of mathematical progression that involves multiplying a common ratio to the previous term. In this article, we will explore the concepts of geometric sequences and series, their applications, and solve some problems related to them. The examples used in this post are not the same as the examples used in the video. You should watch the video first to fully understand what geometric sequences and series are, and then read the following post to consolidate your understanding.
Geometric Sequences
A geometric sequence is a sequence of numbers that follow a particular pattern of multiplication by a constant ratio. The sequence is formed by multiplying each term of the sequence by a constant ratio to obtain the next term. A geometric sequence can be written in the general form as:
a_n = a_1 \cdot r^{n-1}Where:
- a_n is the nth term of the sequence
- a_1 is the first term of the sequence
- r is the common ratio between each term of the sequence
For example, consider the geometric sequence 2, 4, 8, 16, 32, … with the first term a_1=2 and the common ratio r=2. Using the formula, we can find the nth term of the sequence:
a_n = 2\cdot2^{n-1}Thus, the 6th term of the sequence is a_6=2\cdot2^{6-1}=64.
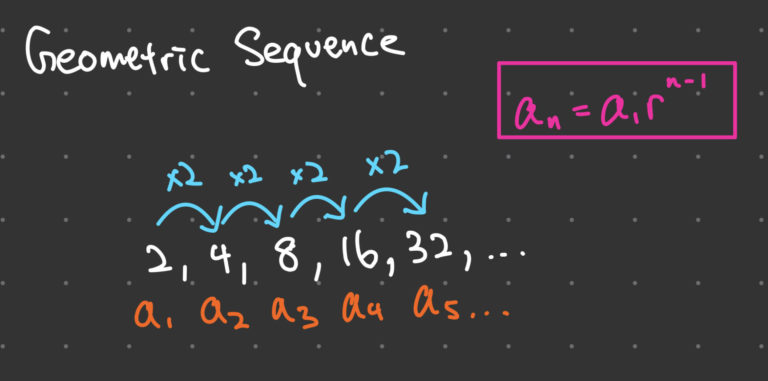
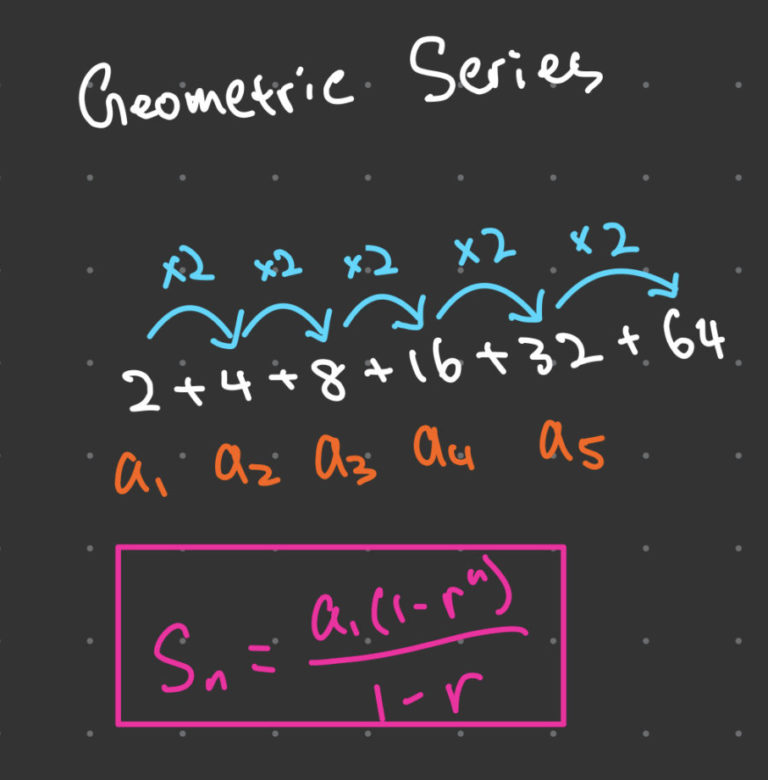
Sum of a Geometric Sequence
The sum of a geometric sequence is known as a geometric series, and can be calculated using the following formula:
S_n=\frac{a_1(1-r^n)}{1-r}Where:
- S_n is the sum of the first n terms of the sequence
- a_1 is the first term of the sequence
- r is the common ratio between each term of the sequence
For example, consider the geometric sequence 2, 4, 8, 16, 32, … with the first term a_1=2 and the common ratio r=2. Using the formula, we can find the sum of the first six terms of the sequence:
\begin{aligned}S_6&=\frac{2(1-2^6)}{1-2} \ &= 2(63) \ &= 126\end{aligned}Thus, the sum of the first six terms of the sequence is 126.
Finding the Common Ratio
To find the common ratio r of a geometric sequence, we can use the formula:
r=\frac{a_{n+1}}{a_n}
Where:
- a_n is the nth term of the sequence
For example, consider the geometric sequence 2, 4, 8, 16, 32, … . To find the common ratio r, we can use the formula:
\begin{aligned}r&=\frac{a_{n+1}}{a_n} \ &= \frac{4}{2} \ &= \frac{8}{4} \ &= \frac{16}{8} \ &= \frac{32}{16} \ &= 2\end{aligned}
Thus, the common ratio of the sequence is 2.
Applications of Geometric Sequences
Geometric sequences have numerous applications in real life, and the ones commonly asked in IB Math exams are listed below:
- Compound interest: The interest earned on a fixed deposit or investment is calculated using a geometric sequence formula. The amount of money earned in each successive year is multiplied by a constant ratio to obtain the total amount earned over the life of the investment.
- Population growth: The growth of populations over time can be modeled using a geometric sequence. Each year, the population increases by a constant ratio to the previous year.
- Growth of bacteria: The growth of bacteria in a culture can be modeled using a geometric sequence. Each hour, the number of bacteria in the culture increases by a constant ratio to the previous hour.
Geometric Series
A geometric series is the sum of a geometric sequence. The sum of the first n terms of a geometric sequence can be calculated using the formula:
S_n=\frac{a_1(1-r^n)}{1-r}
Where:
- S_n is the sum of the first n terms of the sequence
- a_1 is the first term of the sequence
- r is the common ratio between each term of the sequence
Infinite Geometric Series
An infinite geometric series is the sum of an infinite geometric sequence. The formula for the sum of an infinite geometric series is:
S_{\infty}=\frac{a_1}{1-r}
Where:
- S_{\infty} is the sum of an infinite geometric series
- a_1 is the first term of the sequence
- r is the common ratio between each term of the sequence
Applications of Geometric Series
Geometric series also have numerous applications in real life, some of which are listed below:
- Electrical circuits: The resistance of an electrical circuit can be modeled using a geometric series. The total resistance of the circuit is the sum of the resistance of each component, which is proportional to the length of the component.
- Present value: The present value of a future payment or investment can be calculated using a geometric series. The amount of money earned in each successive year is discounted by a constant ratio to obtain the present value.
- Amortization: The amortization of a loan or mortgage can be modeled using a geometric series. The amount of money paid each period is proportional to the remaining balance, which decreases by a constant ratio each period.
Conclusion
Geometric sequences and series are essential topics in the IB Math curriculum. They are widely used in many real-life situations, including compound interest, population growth, and electrical circuits. In this article, we have covered the basics of geometric sequences and series, including their formulas, finding common ratios, and applications. By understanding these concepts, students can solve problems related to geometric sequences and series and apply them in real-life scenarios.
FAQ
What is a geometric sequence?
A geometric sequence is a sequence of numbers that follows a particular pattern of multiplication by a constant ratio. The sequence is formed by multiplying each term of the sequence by a constant ratio to obtain the next term.
What is a geometric series?
A geometric series is the sum of a geometric sequence.
How do you find the common ratio of a geometric sequence?
To find the common ratio of a geometric sequence, you can divide any term of the sequence by the previous term. The result will be the constant ratio between each term of the sequence.
What are some applications of geometric sequences and series?
Geometric sequences and series have numerous applications in real life, including compound interest, population growth, growth of bacteria, electrical circuits, present value, and amortization of loans or mortgages.
What is the formula for the sum of the first n terms of a geometric sequence?
The formula for the sum of the first n terms of a geometric sequence is:
S_n=\frac{a_1(1-r^n)}{1-r}
Where S_n is the sum of the first n terms of the sequence, a_1 is the first term of the sequence, and r is the common ratio between each term of the sequence.
What is an infinite geometric series?
An infinite geometric series is the sum of an infinite geometric sequence. The formula for the sum of an infinite geometric series is:
S_{\infty}=\frac{a_1}{1-r}
Where S_{\infty} is the sum of an infinite geometric series, a_1 is the first term of the sequence, and r is the common ratio between each term of the sequence.
What is the difference between a finite and an infinite geometric series?
A finite geometric series is the sum of a fixed number of terms in a geometric sequence, while an infinite geometric series is the sum of an infinite number of terms in a geometric sequence.
What is the relationship between geometric sequences and exponential functions?
Geometric sequences and exponential functions are related because a geometric sequence can be written in exponential form. The general form of an exponential function is y=a \cdot b^x, where a is the initial value and b is the growth factor. The formula for a geometric sequence is a_n=a_1 \cdot r^{n-1}, where a_1 is the first term of the sequence, r is the common ratio, and n is the term number.